Before the end of Term 1, I attended the #Christmaths event that @mathsjem organised up in London. Unfortunately, I was a bit late getting there and so missed a few of the presentations, but did manage to catch @Kris_Boulton's presentation. He got me thinking about how I taught Trigonometry and whether this was the right approach/best approach to it. What Kristopher said made a lot of sense...he suggested that the way in which we teach topics makes a big difference to whether students really understand what they are being asked to do or whether they have just temporarily learnt a process/method to follow, which is often later forgotten, leading to you having to teach the topic all over again.
Our Y11s had their mock examinations before Christmas and I had marked these before the #Christmaths event and so knew that my Y11s hadn't answered correctly the trigonometry question that was on their paper(s), despite being taught it last year, and most didn't even attempt the question...nothing. So, when I heard Kristopher talking, I thought about how I had taught them Trig and what I could do next time when teaching it to my current Y10s so the topic sticks next time. Kristopher was discussing about how we shouldn't be re-teaching topics every school year and that if we taught it 'right' the first time round, there wouldn't need to waste time re-teaching the topic(s). I know I'm going to have to go over trig with my Y11s again, before their actual GCSEs, so I decided to try my best to improve how I teach this topic to my Y10s so I'm not in the same position next year.
When planning the lessons, I've also incorporated some of the changes to the NEW 1-9 GCSE and I've made the lessons suitable for Foundation students too, when I need to cover this with them (probably, later on in their Y11 year). Initially, though, I have taught the below unit of work/series of lessons to my Higher set 2 Y10s.
Here's my new approach/how I've tweaked things...
This resource is available FREE on my TES resources if you think it would be useful when teaching the topic yourself! See here: https://goo.gl/eR1iNO
The lessons are all on SMART Notebook and on each slide I have 'pull tabs' that allowed me to refer back to learning outcomes, the trig formulae and other self assessment activities at the end of a series of tasks/questions.
I start the series of lessons/unit of work by getting students to measure lengths of similar right-angled triangles and divide pairs of these lengths by each other and see what they find. I then held a discussion with the class as to what they found and why the numbers come out the same, what this means, etc.
After I have discussed this, the ratios between different side lengths being the ratios, sin, cos and tan I then got the students to just focus on labeling the sides of right-angled triangles, dependent on where the angle is.
![]()
After they had comfortably understood the labeling of the sides I then gave the class some examples of how to find the missing length of a right-angled triangle using the sin ratio. During the examples, I refer back to the measuring task at the start of the lesson, bring up my Casio calculator emulator to discuss the importance of typing in the calculations correctly (using brackets), used the SOH triangle and linked to SDT/physics lessons and even referred to the sin graph. I just drew this on the board when a question cropped up about what sin (34) or sin (27) was...I drew the graph and wrote the 90, 180, 270 and 360 angles on the x axis and then drew a line up to the 'wave' and across to the axis to roughly show the value of it, we checked it on the calculator, etc.
![]()
All of the above additions/discussions continued or came up when students were then answering questions themselves.
These slides had 6 questions on them, 3 finding the opposite length and 3 finding the hypotenuse so they'd have to use the SOH triangle both 'ways'.
I got students to round to 3sf at all times as this is something we had covered previously in the year and I wanted them to continue practising this skill as the questions often ask for this degree of accuracy.
There were plenty of opportunities to discuss the rounding to 3sf too, when the answers were, say 8.99542 and the answer would end up 9.00, or when 8.596 came up as the answer and they had to round to 8.60...when they included 0s, when they didn't consider them 'significant', etc.
![]()
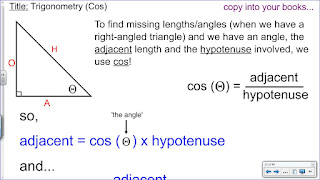
When introducing COS, after having covered finding a missing angle using SIN in a similar way; with me giving examples, showing them the emulator on the board and typing in the calculations, why we use 'shift SIN' and what that meant (what the inverse function was), etc...again drawing back to the graph and showing certain values on here, I gave students some basic notes to copy into their ex books. They had a similar set of notes to write for SIN.
![]()
After covering the slides/lessons on SIN and COS, finding both missing lengths and angles I did a plenary style task whereby students had to identify whether to use SIN or COS - I found students were discussing why it could/couldn't be one of them based on what they were shown quite a bit here and they were convincing each other whether they should or shouldn't stand up.
![]()
I then gave them more practice questions, but this time they had to decide which of SIN/COS to use and whether they were finding opposite, adjacent or hypotenuse. I used the 'pull tabs' lots here, referring back to the formulae for each.
![]()
The NEW 1-9 GCSE includes students knowing exact trig identities, so at the start of one lesson I just put all the trig values students need to know on the board and asked them to write down exactly what came up on their calculator (not to press the S->D button)!
After revealing the answers I dropped the bombshell that they had to remember each of these and be able to recall them in their actual GCSE (just like their times tables)! I said we'd do a timestablesesque quiz soon to test their memory of these. I wrote them on the board so that they could see a pattern between the values. By putting SIN, then COS, SIN, then COS from 0 degrees to 90 degrees you get a pattern emerge - see the slide. I said as long as they remember the first 5 they just reverse the order of the answers for the 2nd 5. As for the TAN values...I just said they'd have to remember these as they were as I didn't see a better way of remembering them!? Has anybody any ways of them remembering these?
In the next set of questions, there was one which comes out as cos-1 (8/16), so cos-1 (1/2) when finding one of the missing angles - at this point I referred back to the trig identities and asked if anyone would know what the answer would be before we even typed it into the calculator, based on what we had done before. Some then had a 'light bulb' moment, shouting out 60 with glee!!
![]()
Once all 3 had been covered, in the same way, keeping the consistency between my approach each lesson so the only thing that was changing was SIN/COS/TAN or what length/angle we were working with, rather than the style of questions, ppt/resource I used, etc, I then gave them a mixture of questions where they had to decide upon what ratio and what they were working out, emphasising that they would not be told which to use in their examinations.
![]()
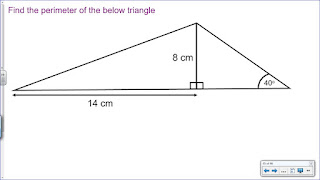
I then gave them some extended problems that used a combination of triangles and needed the use of Pythagoras or Trig.
That's where I'm up to now. I have only just (after 5 lessons) mentioned SOHCAHTOA and have set them the homework task on the resource to find a suitable mnemonic for them to figure out which one to use for any question they're asked.
![]()
Next...I plan on giving them more basic practice questions where they have to decide which to use/work out. Then, I will be giving them some contextualised questions include bearings and combinations of triangles using a different set of resources I have used in the past - just a worksheet of 'wordy' questions. As I understand it...Foundation students will be given a 'simple' type question where they are merely given a right-angled triangle and asked to find a missing length or angle. The 'wordy' contextualised questions with other topics combined with them will be saved for the Higher tier?!
So, I did manage at one point to refer back to our work on Surds and hinted at the fact they may get you to use the trig identities in surd form to calculate a missing side/angle and put your answer in surd form. This went slightly over their heads and may have been too much at that point in that lesson when they had only just been told about knowing these 'off the top of their heads'!
I felt much more confident lesson to lesson when teaching the topic this time round. I thought more about how I was teaching it as I went through the lessons and covered many more questions/misconceptions as I previously had as the students weren't just given SOHCAHTOA in the first lesson and told a method/process to follow as I may have done in previous years...basically just teaching them how to answer a question, without much understanding of what/why they were doing what they were told.
I will see, soon, whether this approach/series of lessons has had an impact. We are following Pearson's 2-year SoW and I'm currently up to Unit 5. In their Unit 5 assessment there are plenty of trig questions that will test their understanding and, of course, in future past papers we give them/specimen papers I'll see if it has 'stuck' this time and hopefully, they won't need teaching it next year.
Please let me know if you've found this useful or have used this resource. There are bits of the resource that I have collated from other teachers...I've used a few of the fantastic Diagnostic Questions from Craig Barton and there are a few slides from other TES users too, which, if they are you (I can't remember who/where I got them from) please let me know so I can give you a mention/shout out here!
Our Y11s had their mock examinations before Christmas and I had marked these before the #Christmaths event and so knew that my Y11s hadn't answered correctly the trigonometry question that was on their paper(s), despite being taught it last year, and most didn't even attempt the question...nothing. So, when I heard Kristopher talking, I thought about how I had taught them Trig and what I could do next time when teaching it to my current Y10s so the topic sticks next time. Kristopher was discussing about how we shouldn't be re-teaching topics every school year and that if we taught it 'right' the first time round, there wouldn't need to waste time re-teaching the topic(s). I know I'm going to have to go over trig with my Y11s again, before their actual GCSEs, so I decided to try my best to improve how I teach this topic to my Y10s so I'm not in the same position next year.
When planning the lessons, I've also incorporated some of the changes to the NEW 1-9 GCSE and I've made the lessons suitable for Foundation students too, when I need to cover this with them (probably, later on in their Y11 year). Initially, though, I have taught the below unit of work/series of lessons to my Higher set 2 Y10s.
Here's my new approach/how I've tweaked things...
This resource is available FREE on my TES resources if you think it would be useful when teaching the topic yourself! See here: https://goo.gl/eR1iNO
The lessons are all on SMART Notebook and on each slide I have 'pull tabs' that allowed me to refer back to learning outcomes, the trig formulae and other self assessment activities at the end of a series of tasks/questions.
I start the series of lessons/unit of work by getting students to measure lengths of similar right-angled triangles and divide pairs of these lengths by each other and see what they find. I then held a discussion with the class as to what they found and why the numbers come out the same, what this means, etc.
After I have discussed this, the ratios between different side lengths being the ratios, sin, cos and tan I then got the students to just focus on labeling the sides of right-angled triangles, dependent on where the angle is.
These slides had 6 questions on them, 3 finding the opposite length and 3 finding the hypotenuse so they'd have to use the SOH triangle both 'ways'.
I got students to round to 3sf at all times as this is something we had covered previously in the year and I wanted them to continue practising this skill as the questions often ask for this degree of accuracy.
There were plenty of opportunities to discuss the rounding to 3sf too, when the answers were, say 8.99542 and the answer would end up 9.00, or when 8.596 came up as the answer and they had to round to 8.60...when they included 0s, when they didn't consider them 'significant', etc.
After revealing the answers I dropped the bombshell that they had to remember each of these and be able to recall them in their actual GCSE (just like their times tables)! I said we'd do a timestablesesque quiz soon to test their memory of these. I wrote them on the board so that they could see a pattern between the values. By putting SIN, then COS, SIN, then COS from 0 degrees to 90 degrees you get a pattern emerge - see the slide. I said as long as they remember the first 5 they just reverse the order of the answers for the 2nd 5. As for the TAN values...I just said they'd have to remember these as they were as I didn't see a better way of remembering them!? Has anybody any ways of them remembering these?
In the next set of questions, there was one which comes out as cos-1 (8/16), so cos-1 (1/2) when finding one of the missing angles - at this point I referred back to the trig identities and asked if anyone would know what the answer would be before we even typed it into the calculator, based on what we had done before. Some then had a 'light bulb' moment, shouting out 60 with glee!!
That's where I'm up to now. I have only just (after 5 lessons) mentioned SOHCAHTOA and have set them the homework task on the resource to find a suitable mnemonic for them to figure out which one to use for any question they're asked.
So, I did manage at one point to refer back to our work on Surds and hinted at the fact they may get you to use the trig identities in surd form to calculate a missing side/angle and put your answer in surd form. This went slightly over their heads and may have been too much at that point in that lesson when they had only just been told about knowing these 'off the top of their heads'!
I felt much more confident lesson to lesson when teaching the topic this time round. I thought more about how I was teaching it as I went through the lessons and covered many more questions/misconceptions as I previously had as the students weren't just given SOHCAHTOA in the first lesson and told a method/process to follow as I may have done in previous years...basically just teaching them how to answer a question, without much understanding of what/why they were doing what they were told.
I will see, soon, whether this approach/series of lessons has had an impact. We are following Pearson's 2-year SoW and I'm currently up to Unit 5. In their Unit 5 assessment there are plenty of trig questions that will test their understanding and, of course, in future past papers we give them/specimen papers I'll see if it has 'stuck' this time and hopefully, they won't need teaching it next year.
Please let me know if you've found this useful or have used this resource. There are bits of the resource that I have collated from other teachers...I've used a few of the fantastic Diagnostic Questions from Craig Barton and there are a few slides from other TES users too, which, if they are you (I can't remember who/where I got them from) please let me know so I can give you a mention/shout out here!